Mixture Problems
amount of substance in the solution = quantity of solution × strength of solution (in percent)
(amount of substance in mixture 1) + (amount of substance in mixture 2) = amount of substance in mixture
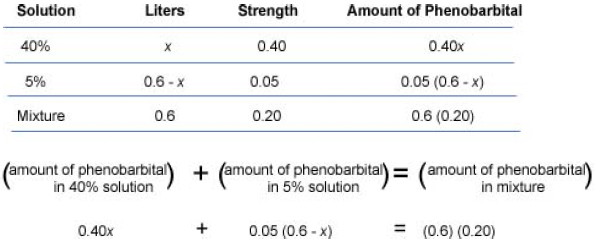
0.40x + 0.05(0.6 − x) = (0.6)(0.20)0.40x + 0.03 − 0.05x = 0.12 distributive property on left; multiply on right0.35x + 0.03 = 0.12 combine like terms0.35x = 0.09 subtract 0.03 from both sidesx = 0.26 divide both sides by 0.35
Practice
Round answers to the nearest hundredth.
1) An orange punch contains 4% orange juice. If 5 ounces of water is added to 8 ounces of the punch, determine the percent of orange juice in the solution.
Use the formulas:
quantity × strength (in percent) = amount of substance
(amount of juice in punch) + (amount of juice in water) = (amount of juice in mixture)
2) The active ingredient in one type of Ortho insect spray is 50% malathion. The instructions on the bottle say to add 1 fluid ounce to a gallon (128 fluid ounces) of water. What percent of malathion will be in the mixture?
3) Suppose the dolphins at Sea World must be kept in salt water with an 0.8% salt content. After a week of warm weather, the salt content has increased to 0.9% due to water evaporation. How much water with 0% salt content must be added to the 50,000 gallons of the 0.9% salt water to lower the salt concentration to 0.8%?
4) Miracle-Gro All Purpose liquid plant food has 12% nitrogen. Miracle-Gro Quick Start liquid plant food has 4% nitrogen. If 12 cups of the All Purpose plant food are mixed with 3 cups of the Quick Start plant food, determine the percent of nitrogen in the mixture.
5) The label on Listerine mint mouthwash says that it is 21.6% alcohol by volume. The label on the Scope mint mouthwash says that it is 15.0% alcohol by volume. If 6 ounces of Listerine are mixed with 4 ounces of Scope, what is the percent alcohol of the mixture?
6) A pharmacist has a 50% solution of sodium iodite. She also has a 25% solution of the same drug. She gets a prescription calling for a 40% solution of the drug. How much of each solution should she mix to make 0.5 liter of the 40% solution?
Answers
1. 2.46%
2. 0.39%
3. 6,250 gallons
4. 10.4%
5. 18.96%
6. 0.3 liters of the 50% solution, 0.2 liters of the 25% solution